Any measurement in chemistry is limited to some extent by two properties: its accuracy and its precision. The goal of any measurement is to obtain the True Value of whatever it is we are trying to measure. The True Value might be the mass of a substance, its volume, the wavelength of light absorbed or emitted from a substance, the intensity of light, or any other measured value. All of science hinges on the understanding that for any measured value, there exists a True Value. Our ability to find it depends on the quality of tool or instrument we use to measure it.

Are you measuring the same mass each time? Yes.
Will the number you get on each scale be the same? Doubtful!
What if you took repeated measurements, would you get the same mass each time with each scale?
Would each scale give you the same number of decimal points?
Accuracy is a measure of how close a measured value is to the True Value.
Precision is a measure of how close repeated measurements are to each other.
Suppose that this archery target represents a given measurement. The bullseye represents the True Value.

Here is an example of a measurement that would be both accurate and precise. All measurements represent the True Value and they are all close to each other.

In this case, the measurements are not precise or accurate. This is by far the worst situation with any type of measurement. Finding the True Value is going to be impossible with a method like this.
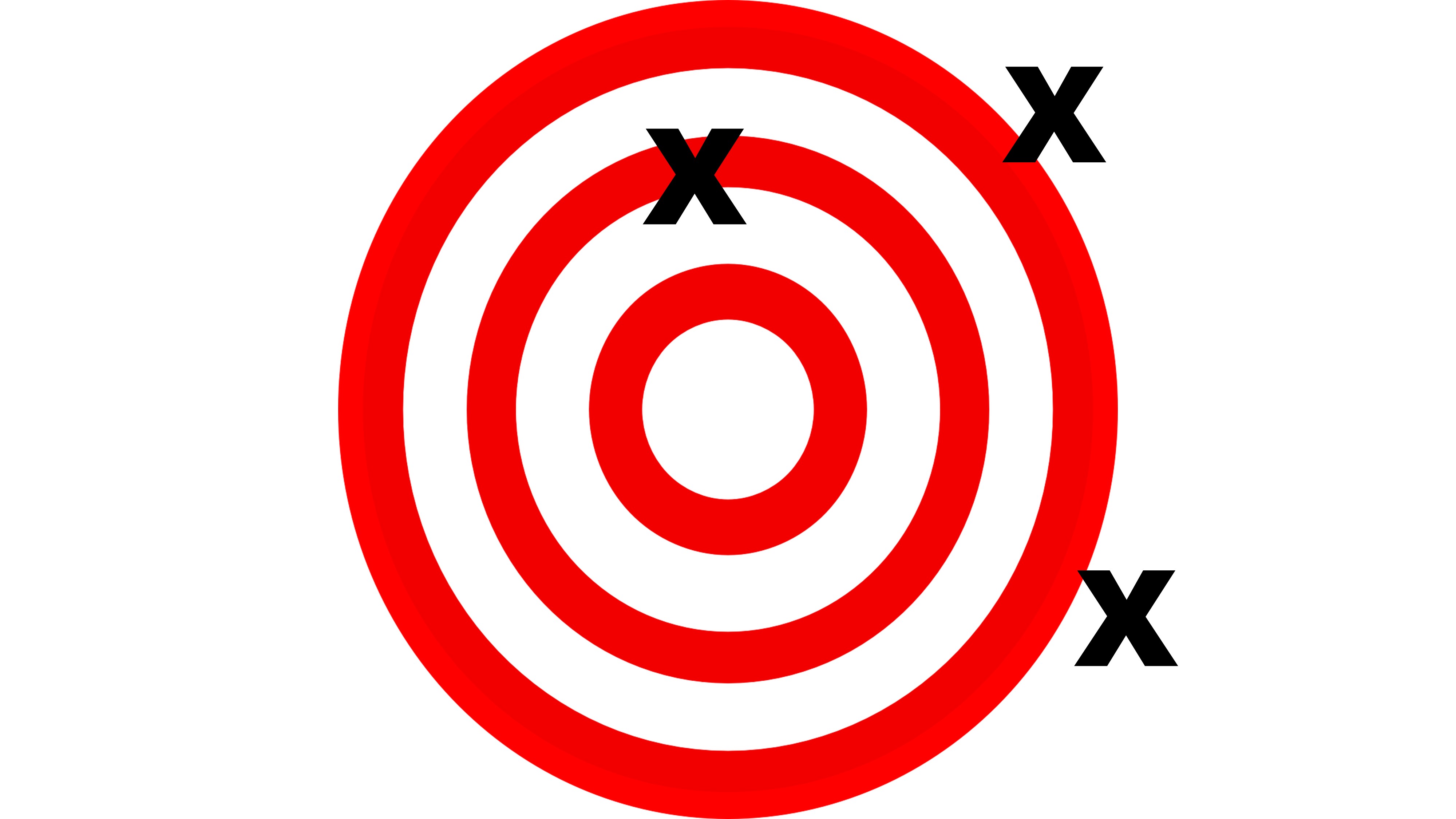
In this case, measurements are accurate, but not precise. The repeated measurements don’t match each other, but the average of the measurements would land on the bullseye.
This type of measurement is how most measurements in science are done. Any individual measurement cannot be trusted, but by repeating measurements several times, an average can be calculated that represents the true value.

In this case, the measurements are precise, but not accurate. Repeated measurements are close to each other, but they do not represent the True Value.
This example would be like a scale with a constant offset, one that is always off but always by the same amount. This type of measurement is easily corrected by simply applying a calibration.
For example, you can easily tell time with a watch that is five minutes fast as long as you always subtract five minutes from the time you read.

